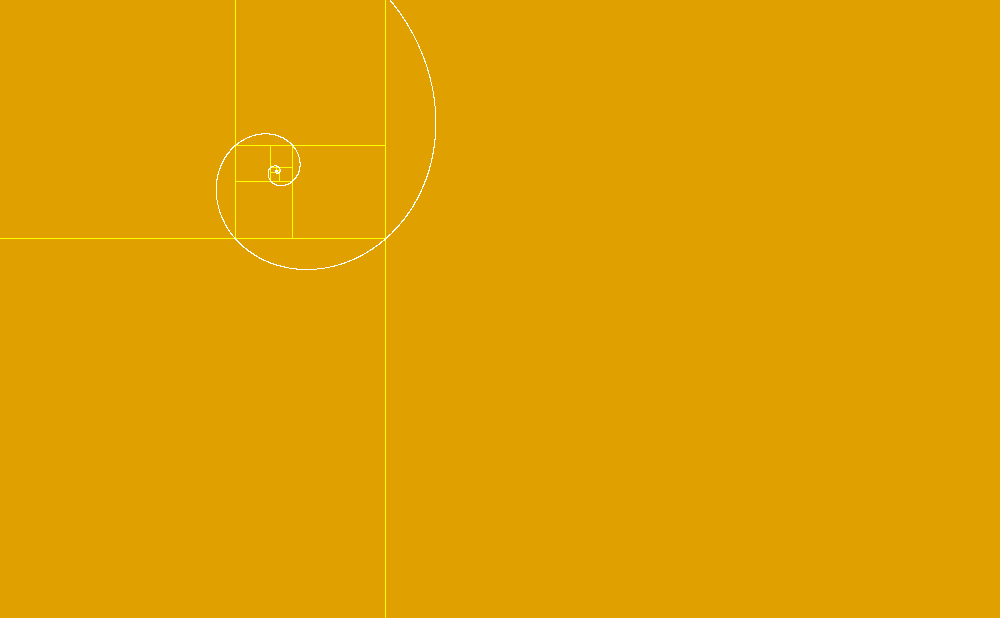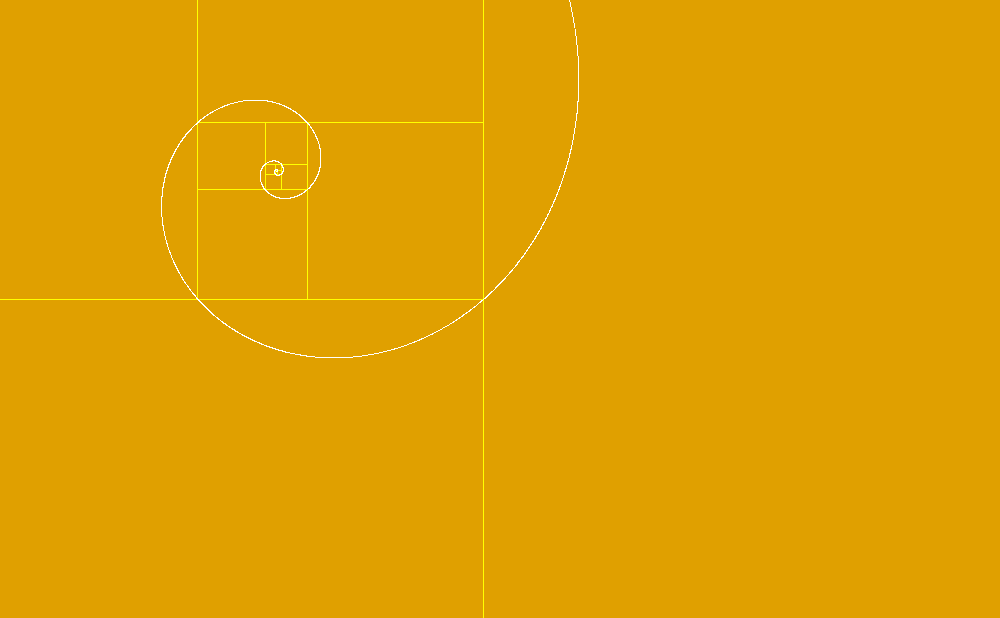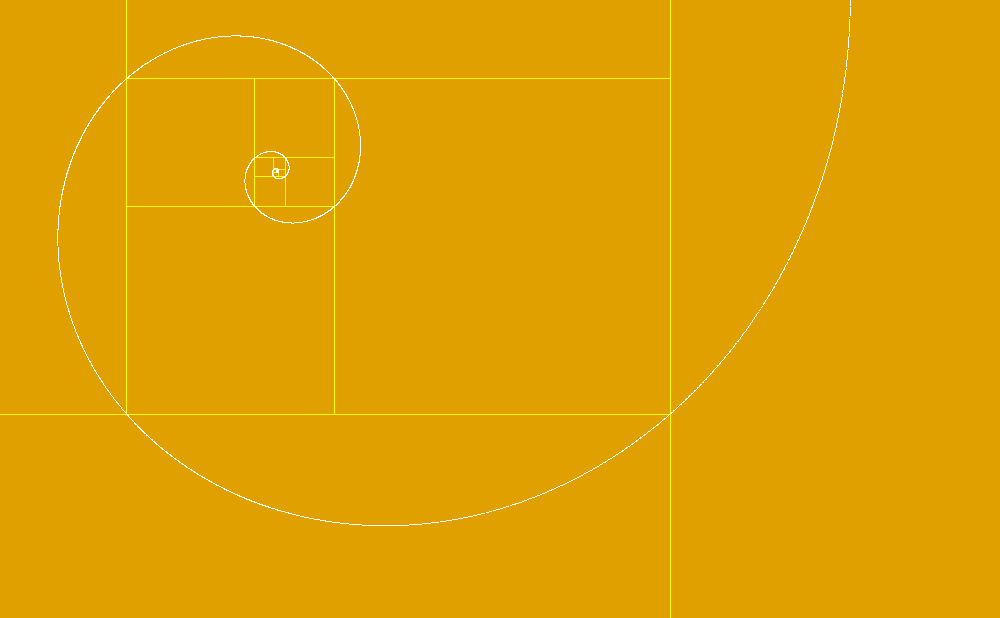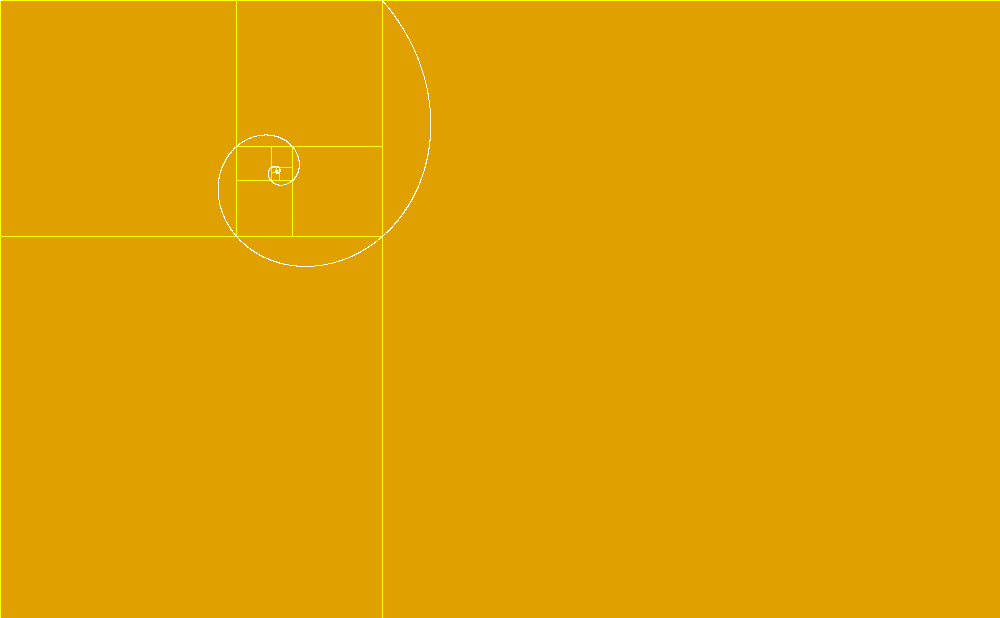Golden rectangle and Fibonacci sequence

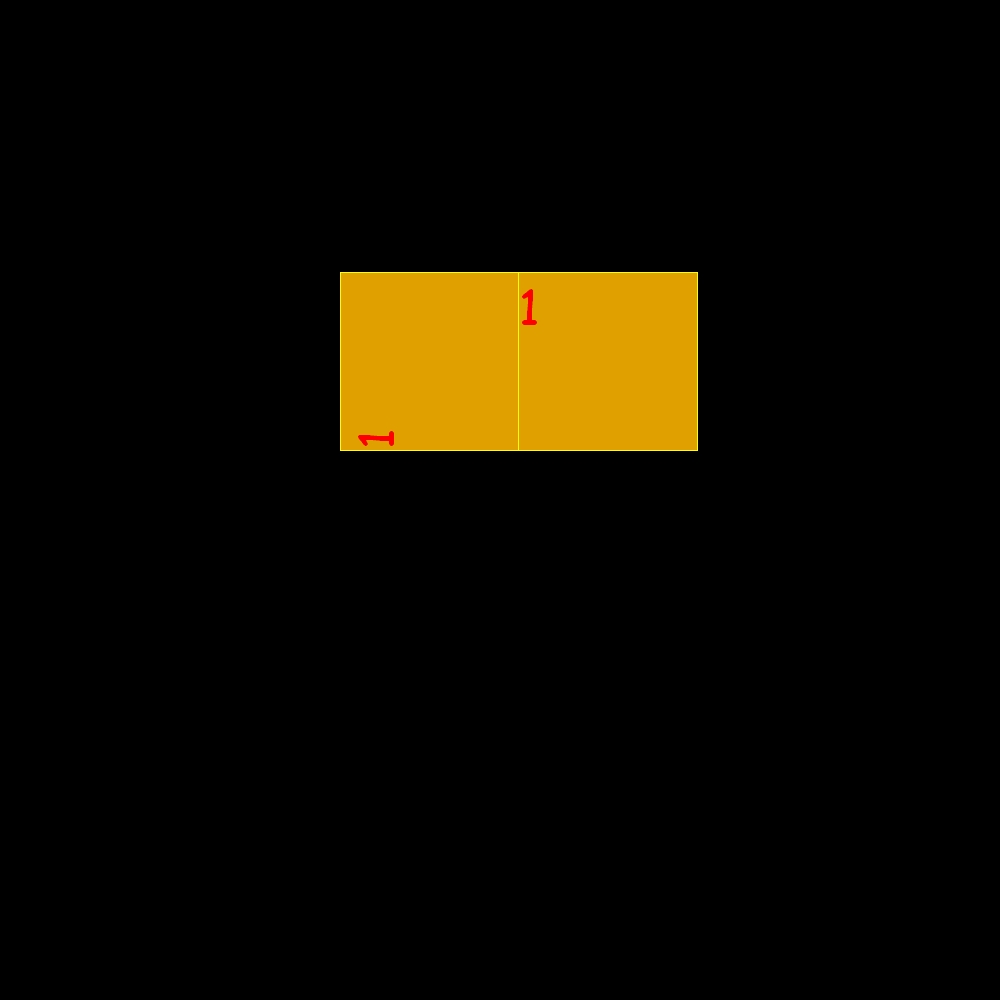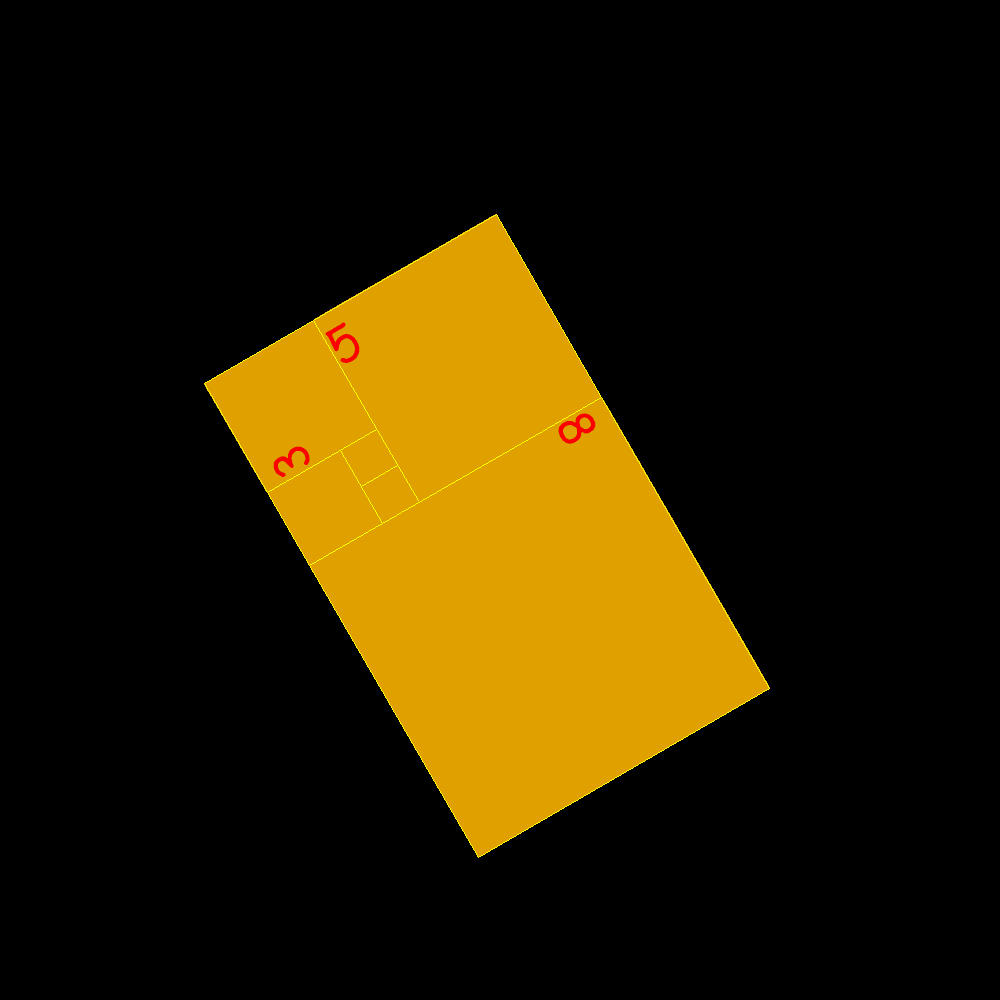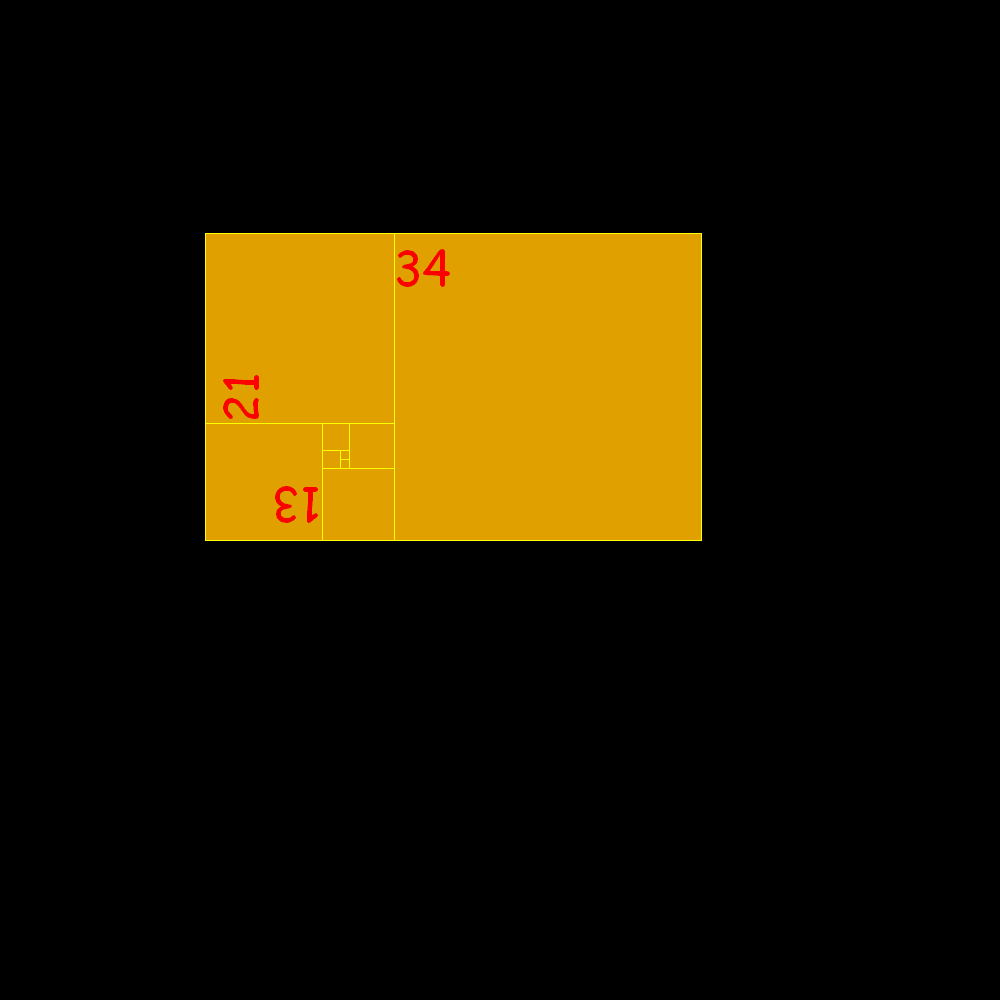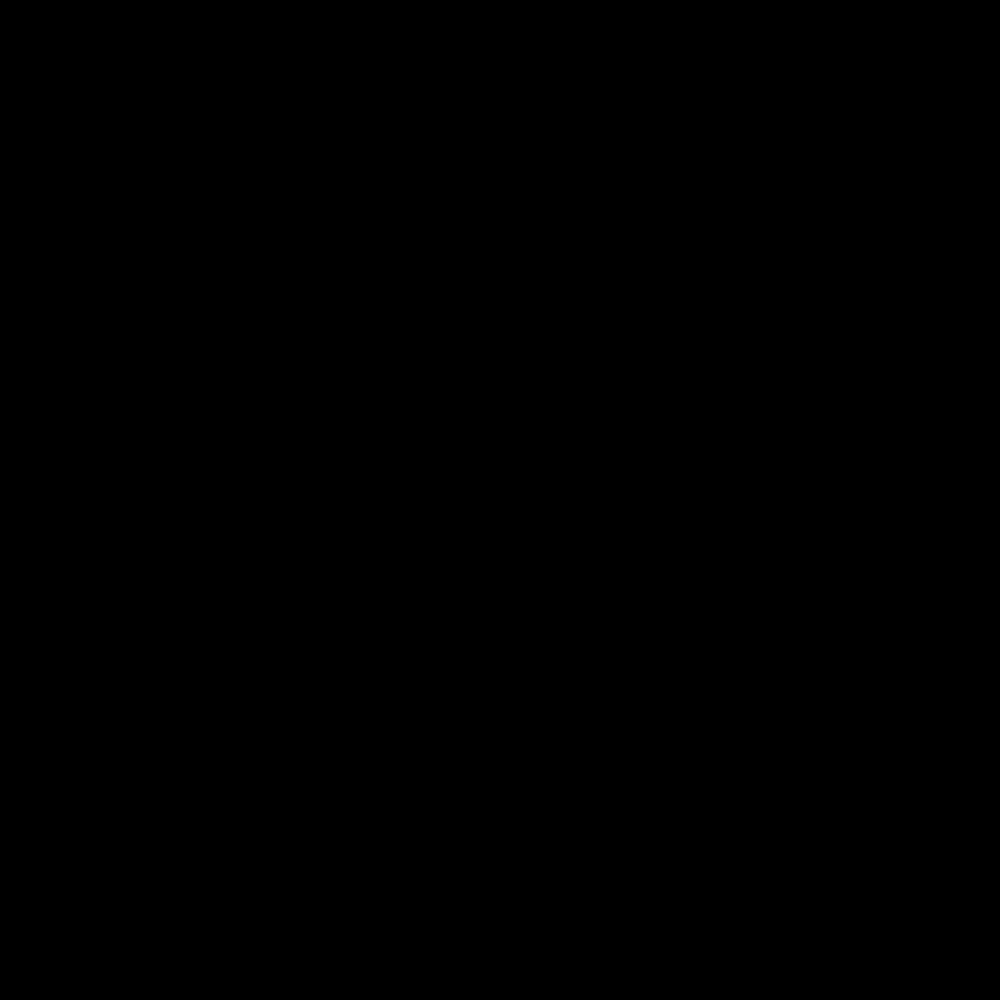
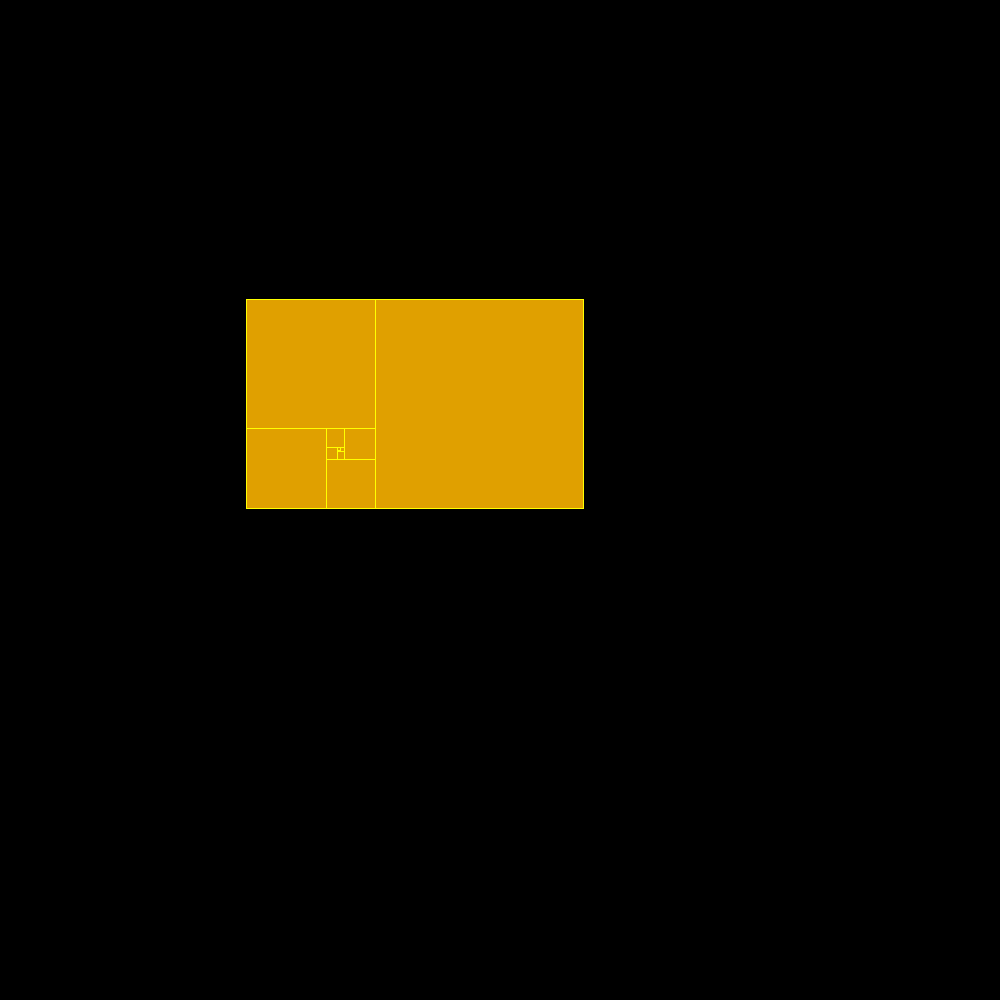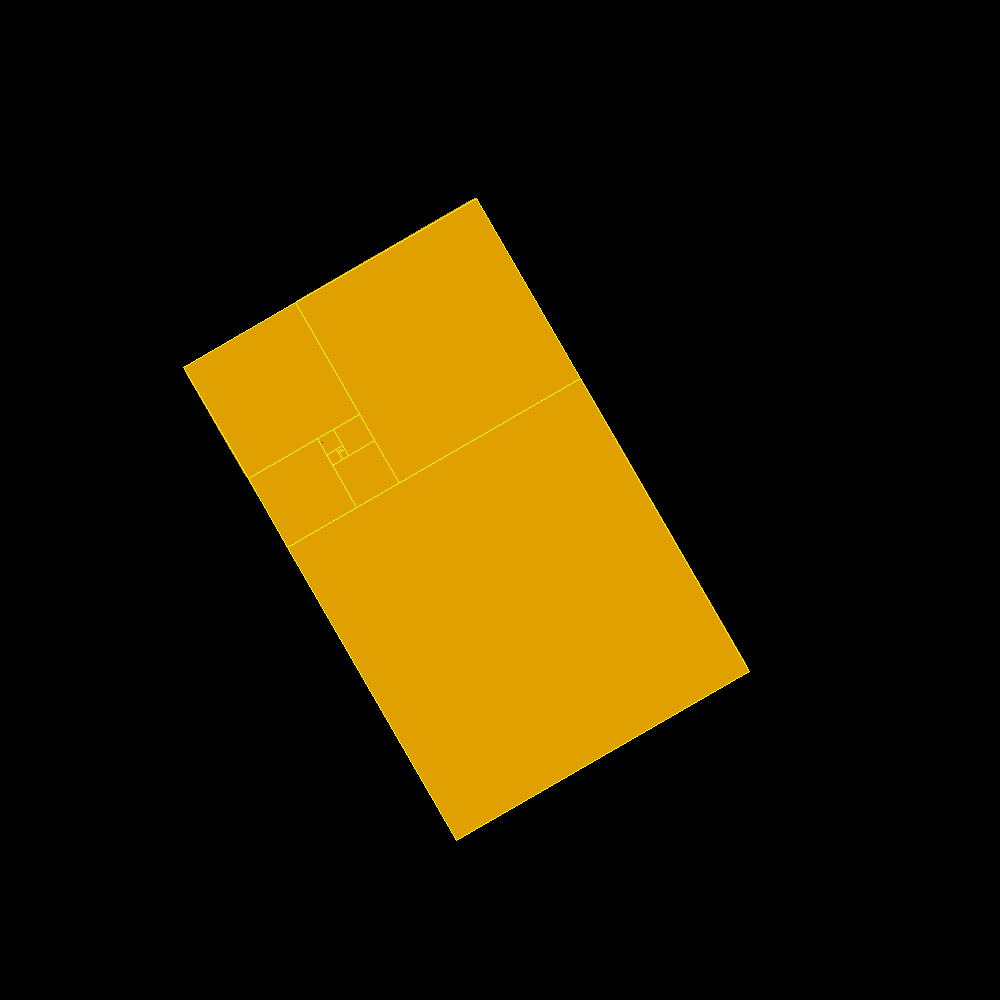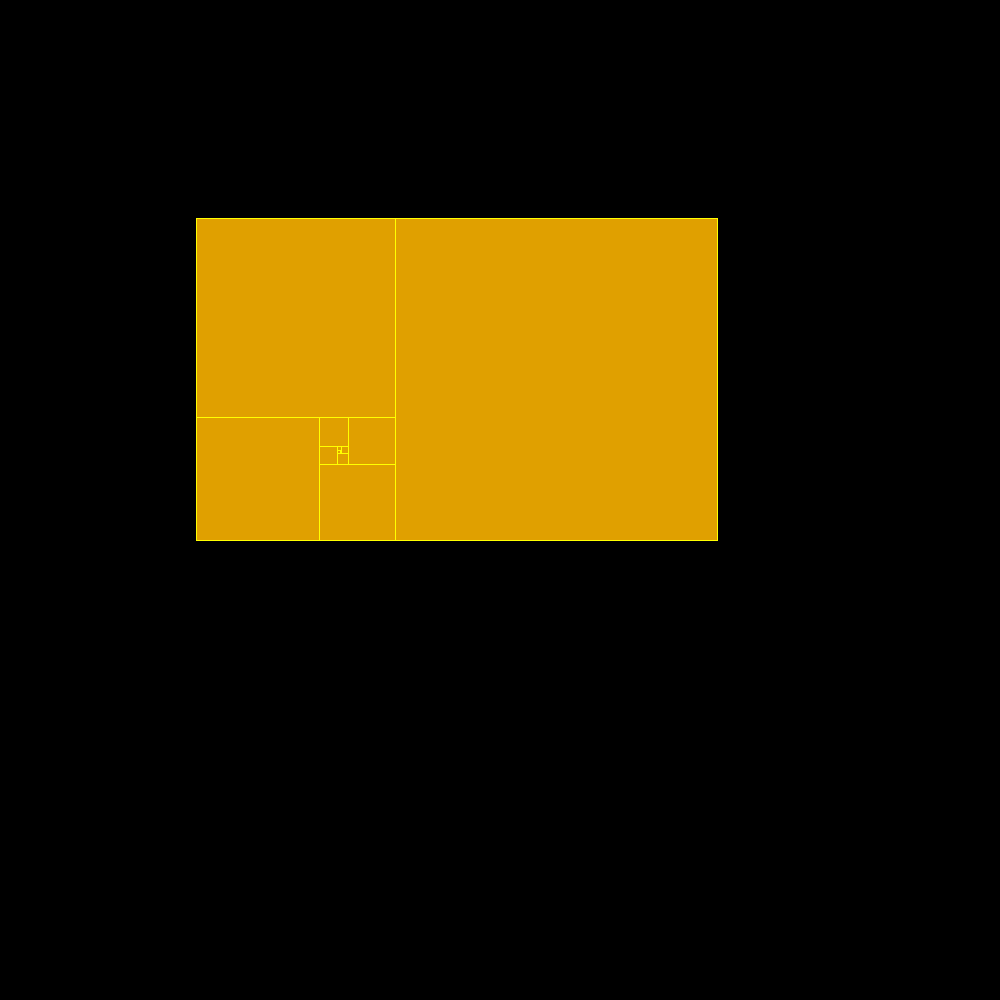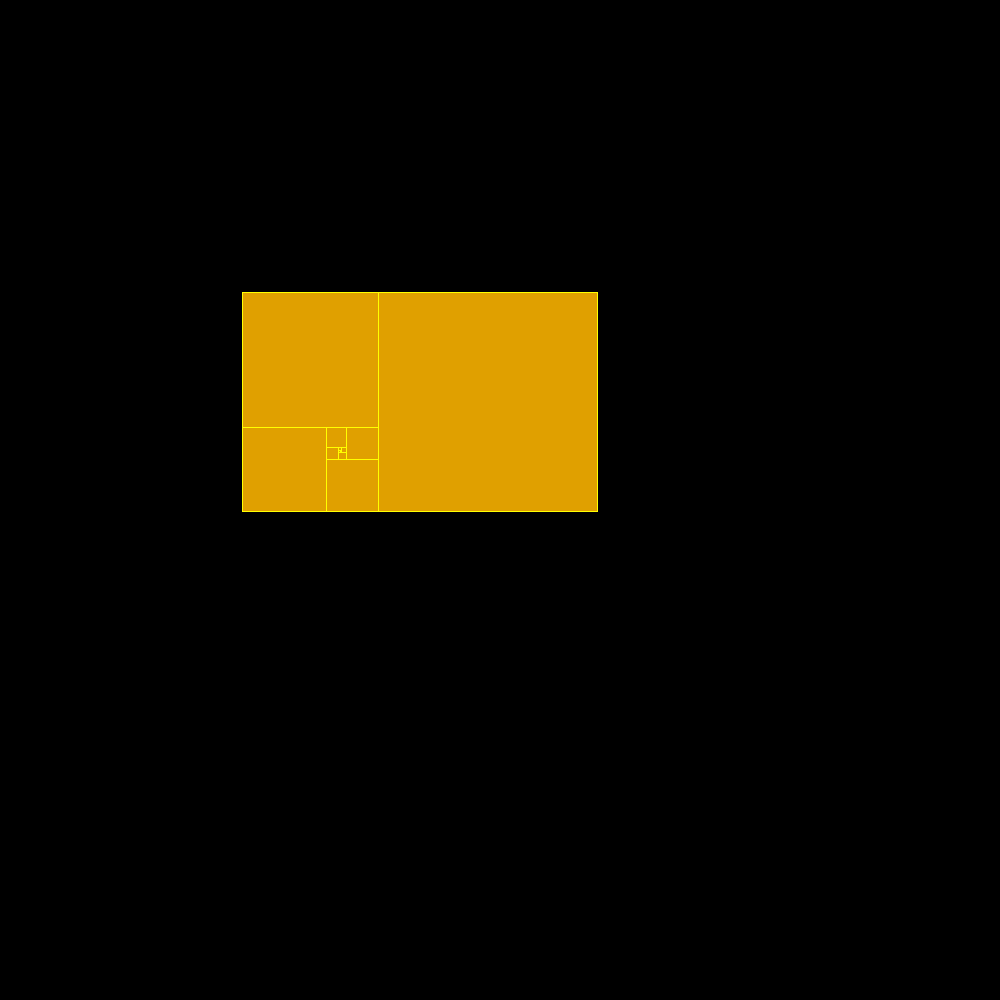
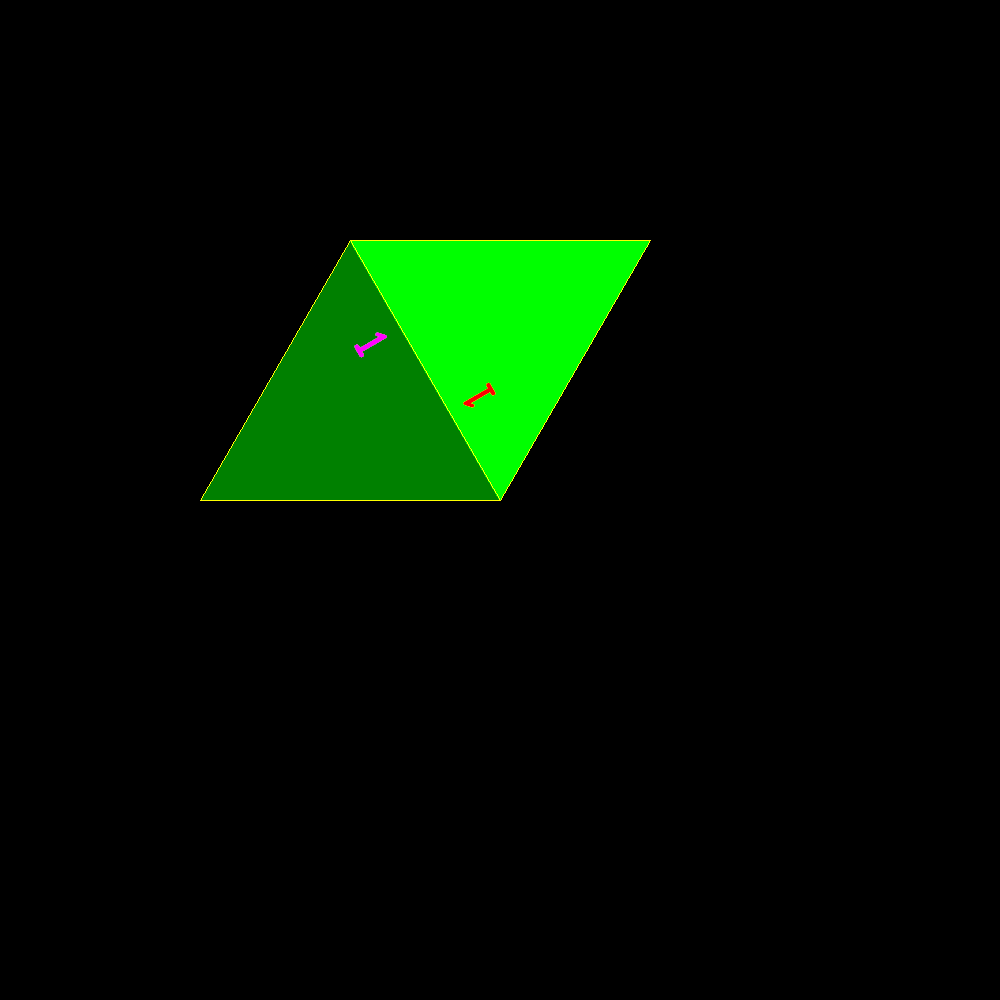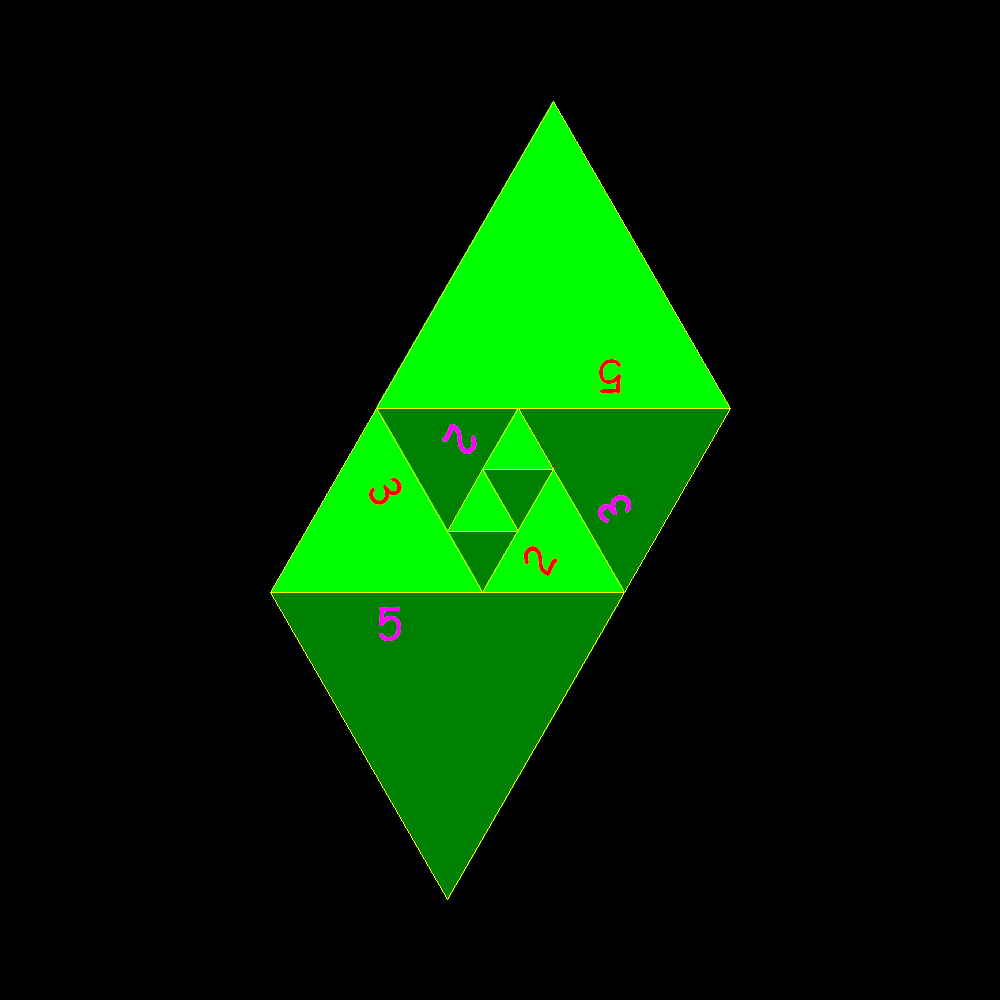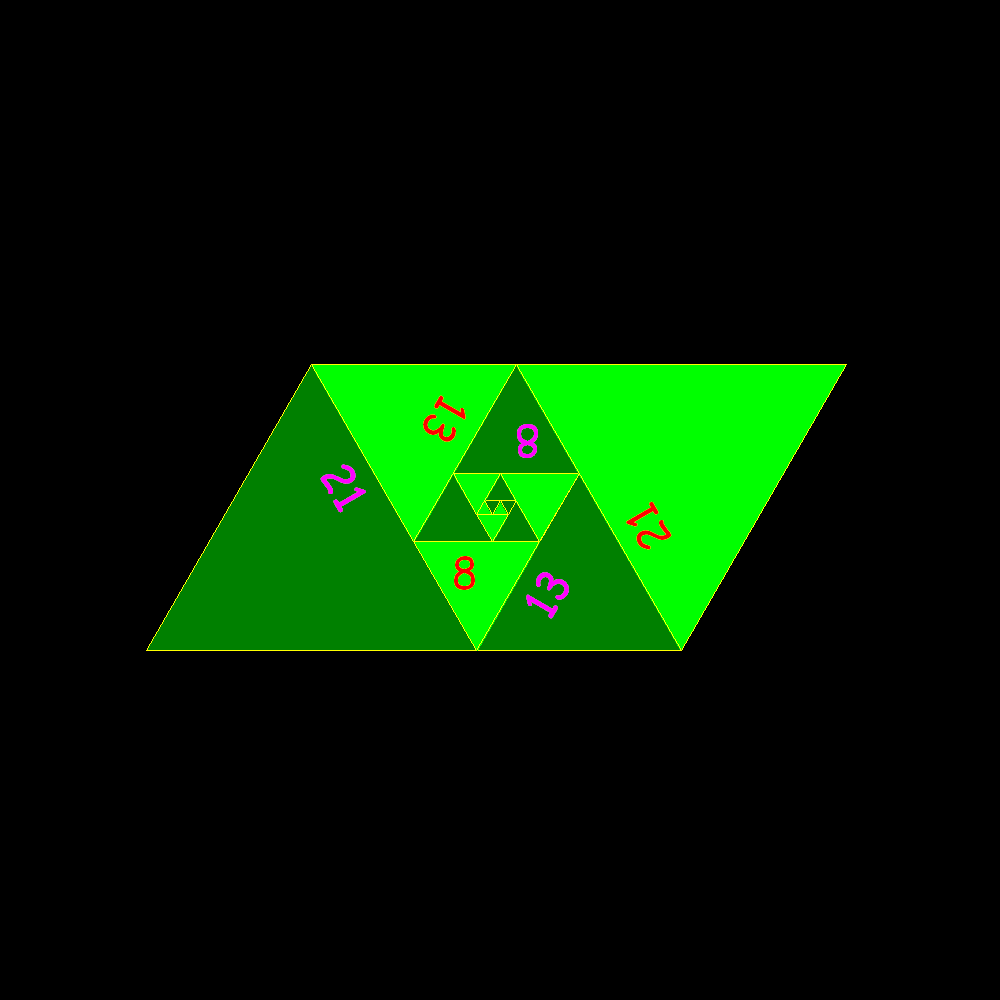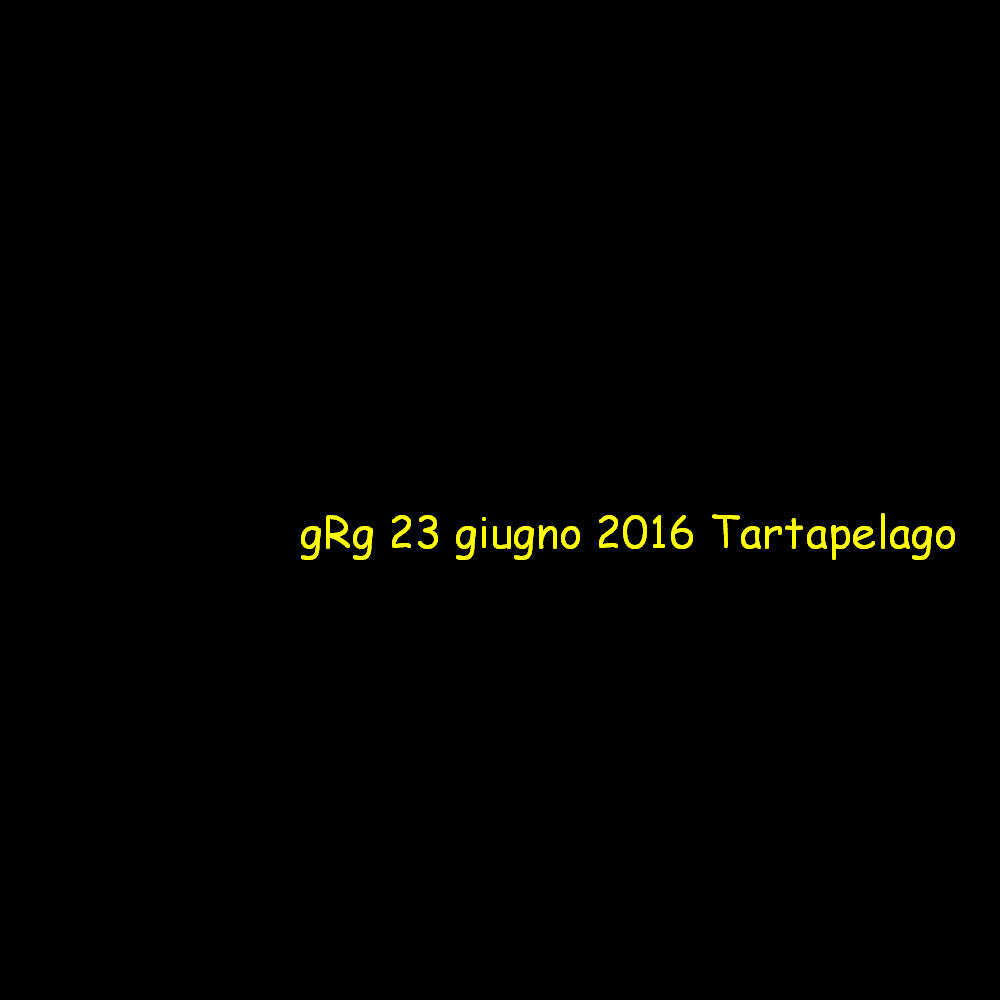
From the rectangle obtained it is observed that the side of a square equals the sum of the two immediately preceding.
For this you can tend to the golden rectangle building rectangles with square in the following sequence:

1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 144,  233, 377, 610, 987, 1597, 2584, 4181, 6765, 10946, ...

that it is the famous Fibonacci numbers with which counted the successive pairs of rabbits


Ratio of two consecutive numbers of the sequence tends to the relationship between major and minor side of the golden rectangle ::

f =1.6180339887499....      
one of the solutions of the following equation:
r²-r-1=0

(1/f=0.6180339887499... one of the solutions of the following equation: r²+r-1=0)

Start of rectangles identified by square building with sides according to the Fibonacci sequence

Shortly after the result is confused with the golden  rectangle to which it tends

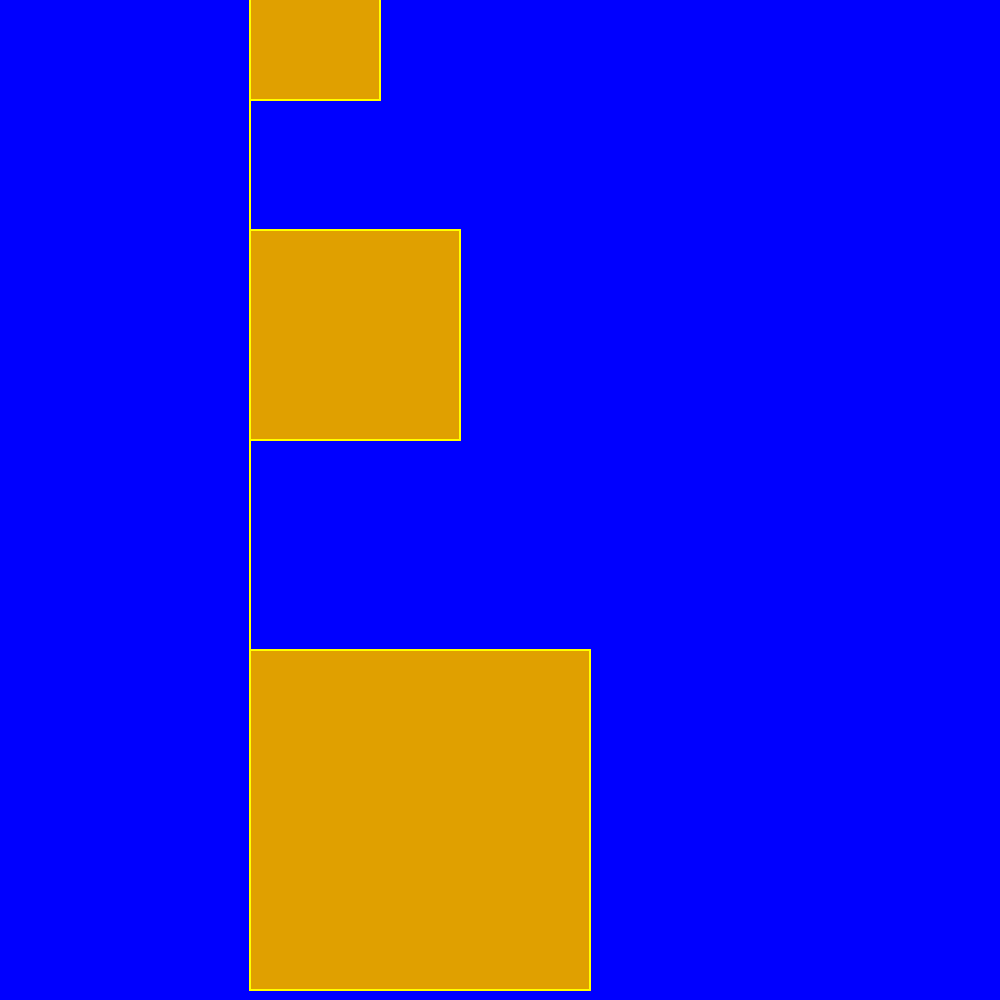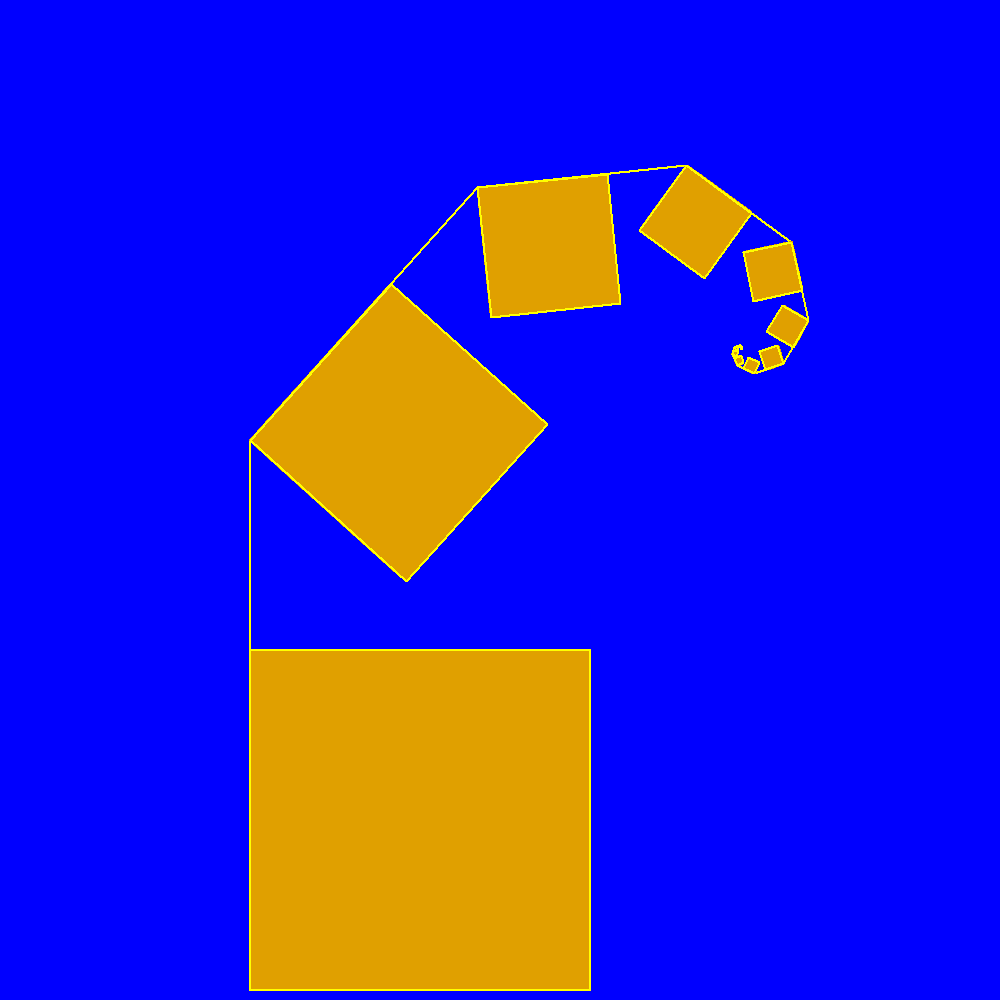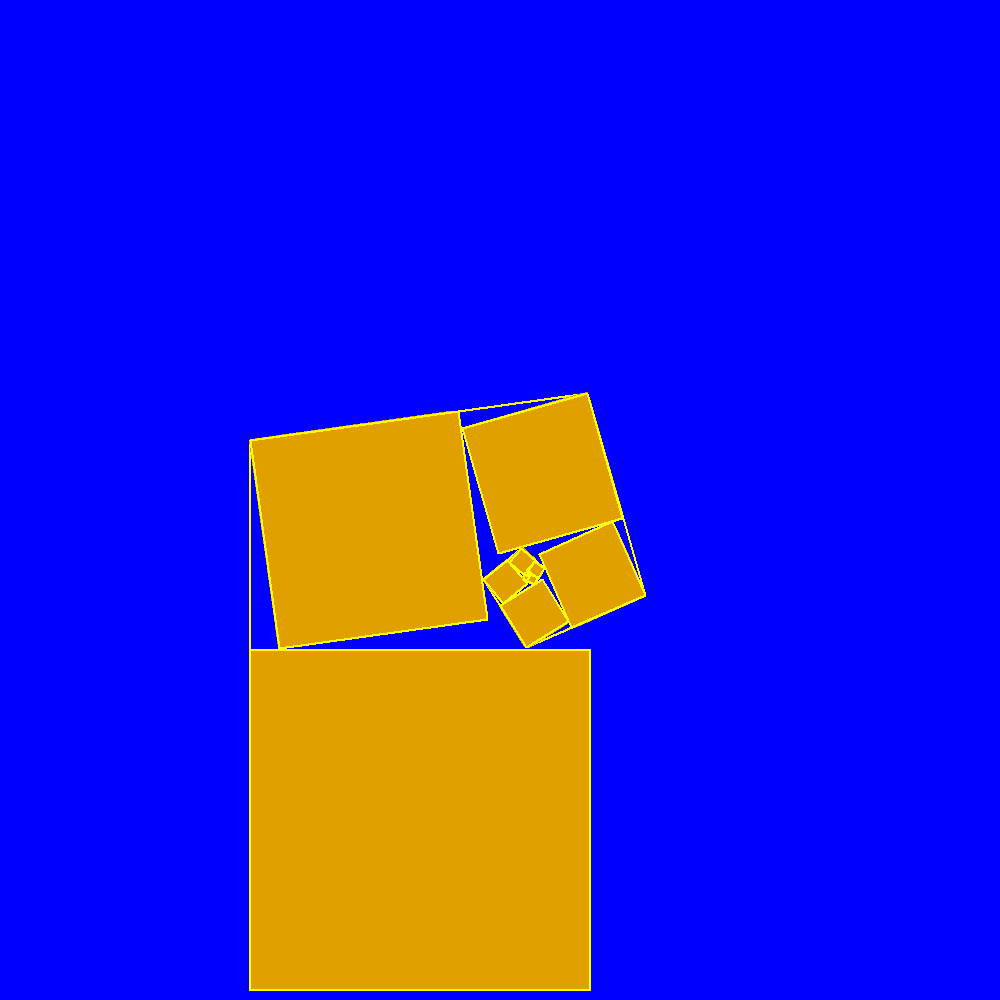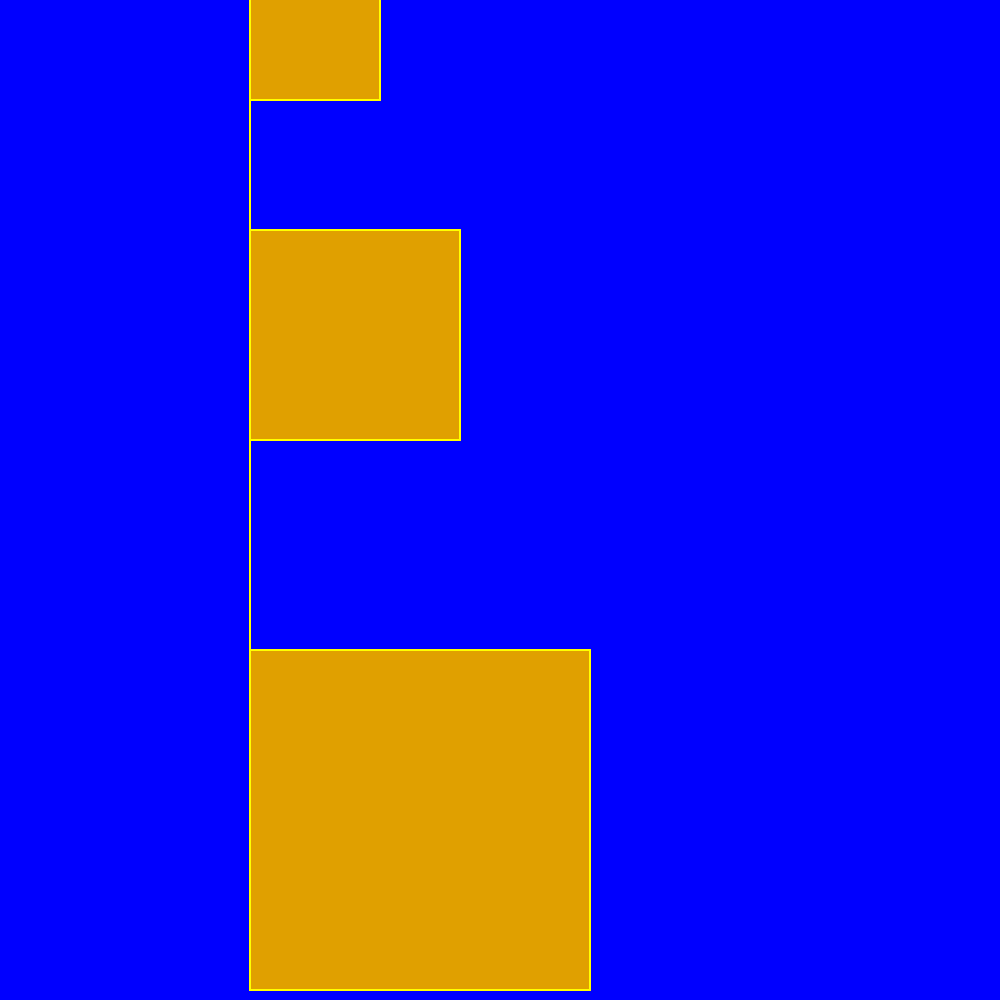packing and unpacking (the first one)

packing and unpacking (the second one)

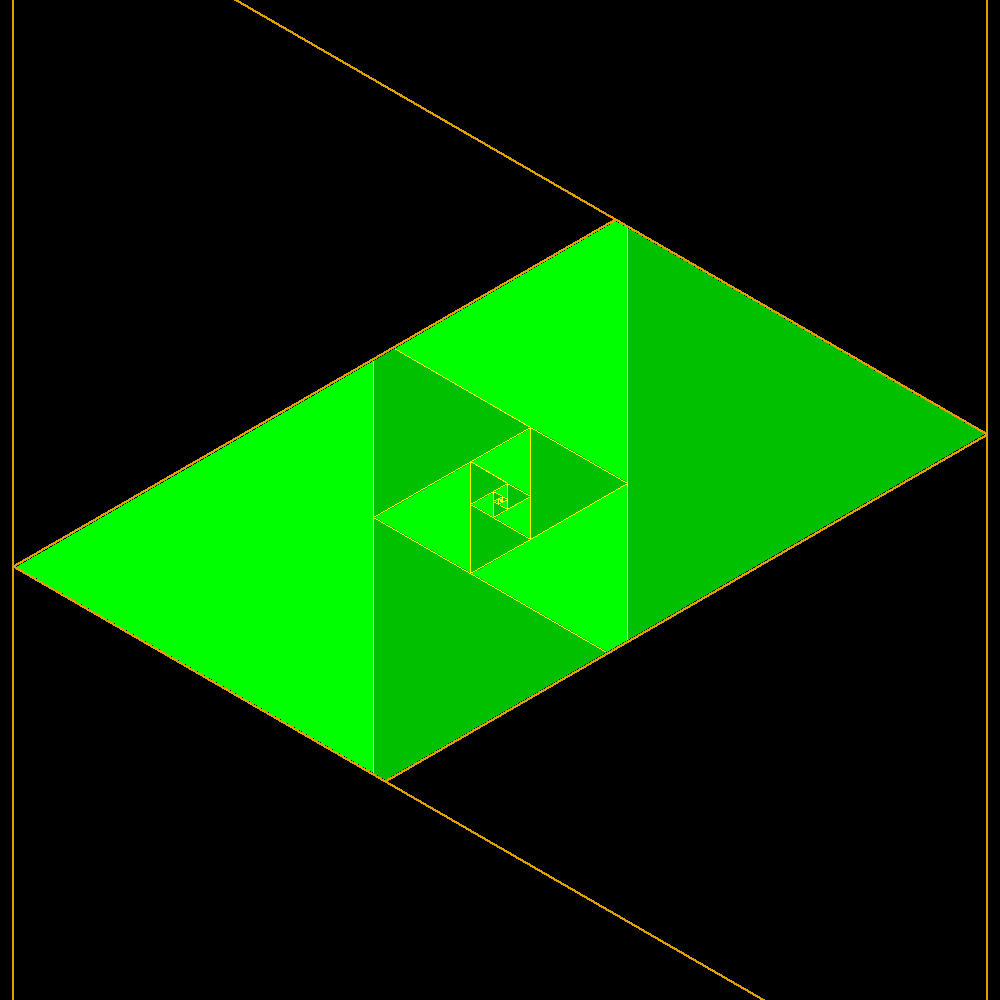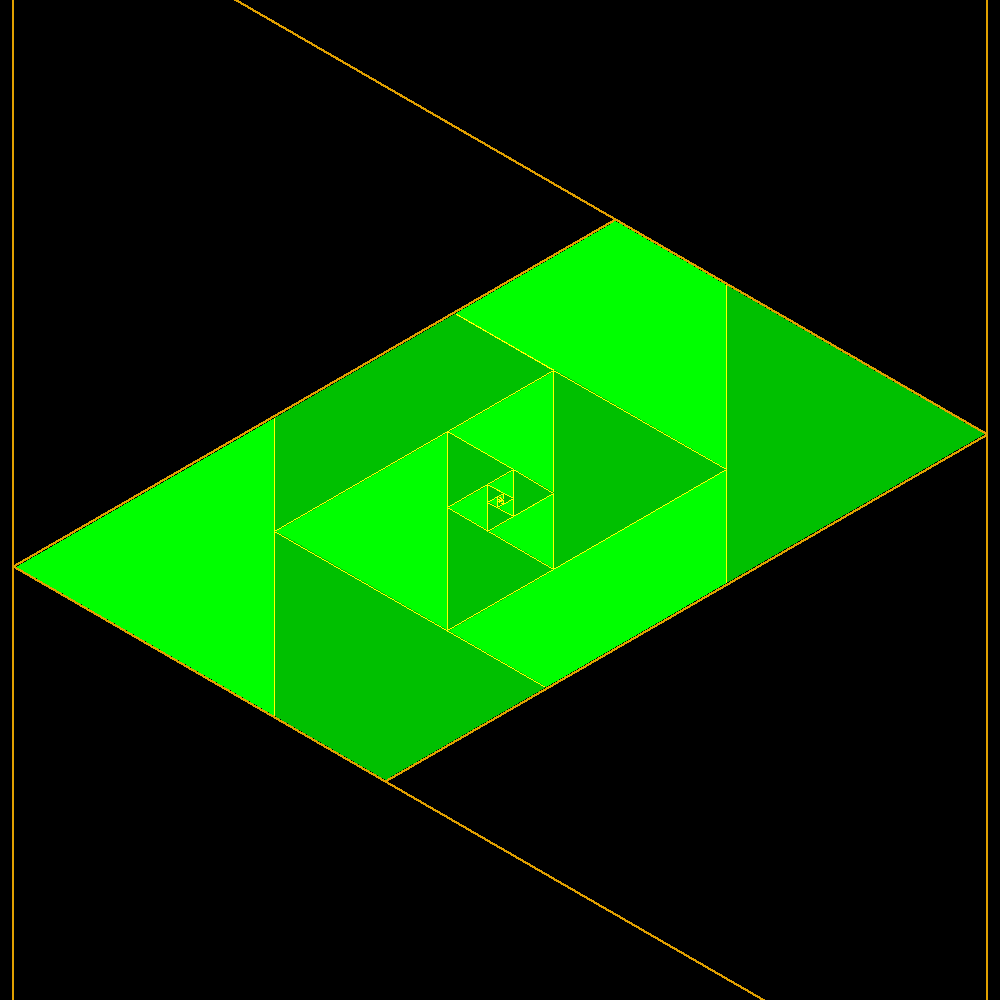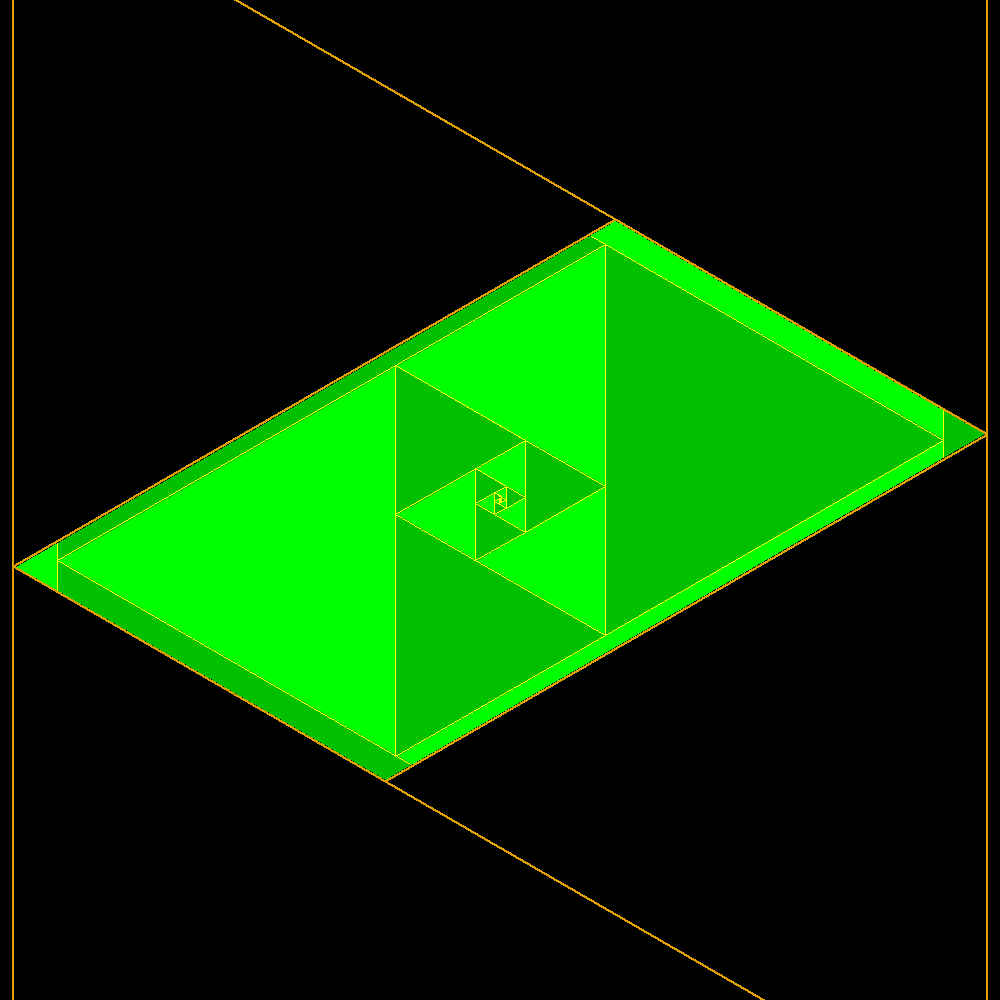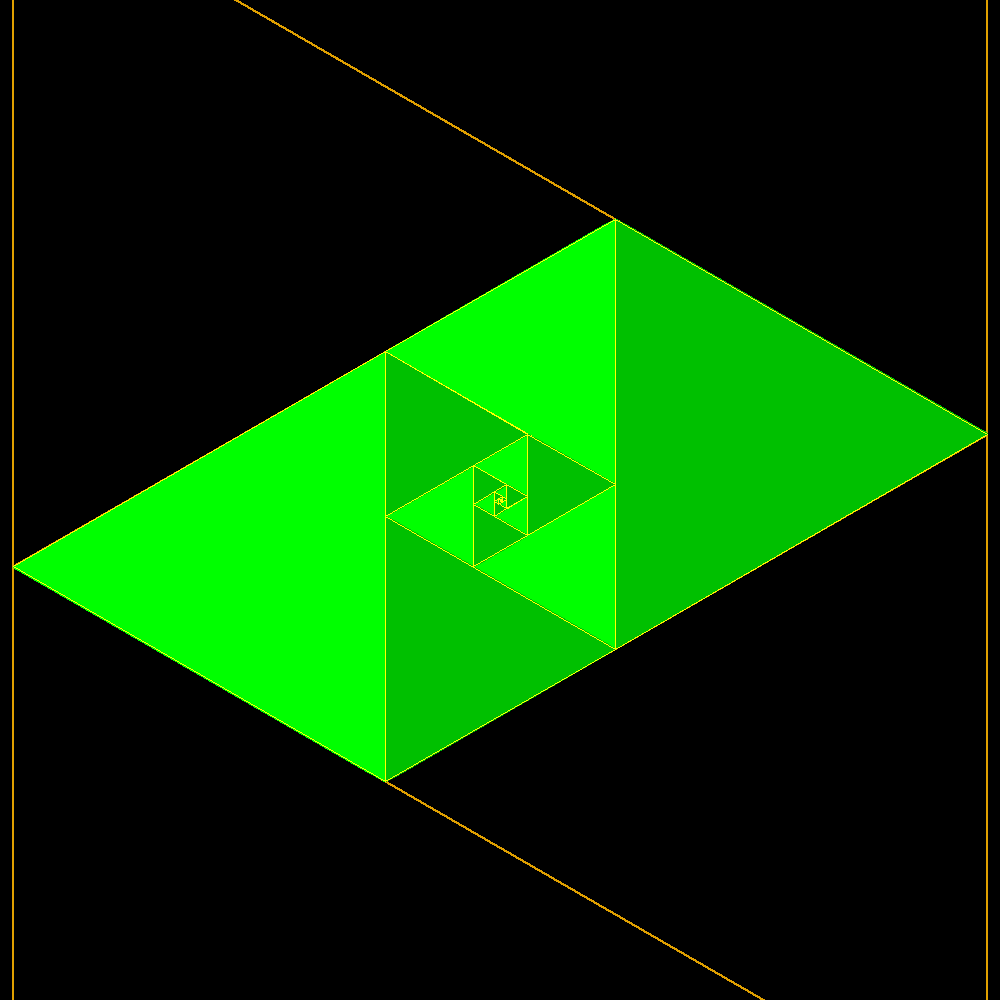
Start of construction of parallelogram with equilateral triangles according with the  Fibonacci sequence

Shortly after the result is confused with the golden parallelogram to which it tends

golden parallelogram

packing and unpacking